[This is post #10 in a mini-blog-post series for NaBloPoMo 2015. Jump to the first, previous, or next post.]
What makes yesterday’s “Hard Mode” so hard? It’s more than just spacial reasoning.
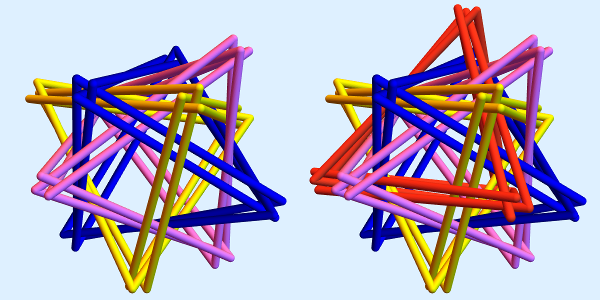
In addition to puzzling out the locations of all the straws, we also need a strategy to keep them where you want them! The images so far have been perhaps a bit misleading. The single tetrahedron I photographed a few days ago?
Yeah, that’s not quite right. Here’s what actually happens when you build this tetrahedron:
It just doesn’t stay put. Indeed, looking more closely at the static image above, you may notice small pieces of tape in the back corner holding things together. Now you know why. Not until very near the end (about 4 out of 5 tetrahedra) are the straws able to hold each other in place without some form of extra support.
So all of you intrepid “Hard Mode” explorers should consider this an amendment to yesterday’s Step 2 (Hard Mode): use some tape, or string, or twist-ties, or dozens of hands[1] to hold things steady as you build.
For the rest of us, on to the actual steps tomorrow!
Notes
- They work better when attached to their owners. [↩]













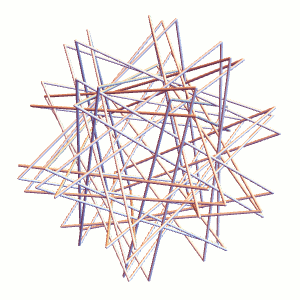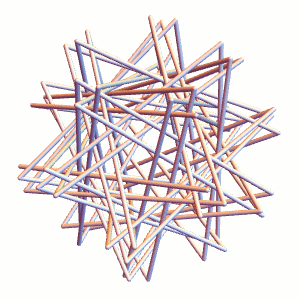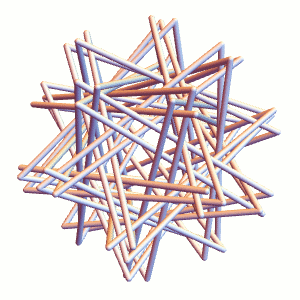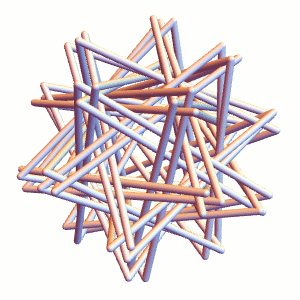











![[Straws Thingy]](http://i1.wp.com/blog.zacharyabel.com/wp-content/uploads/2016/05/straws-thingy-480.jpg?resize=480%2C480)
![[up&up Straws from Target]](http://i0.wp.com/blog.zacharyabel.com/wp-content/uploads/2015/11/up-and-up-straws.jpg?resize=480%2C360)